Related Resources: beam bending
Double Integration Method Example 1 Simply Supported Beam with Concentrated Load at Mid Spa
Beams Deflection and Stress Formulas and Calculators
Engineering Mathematics
Double Integration Method Example Proof Simply Supported Beam of Length L with Concentrated Load at Mid Span
The Double Integration Method, also known as Macaulay’s Method is a powerful tool in solving deflection and slope of a beam at any point because we will be able to get the equation of the elastic curve.
Elastic Curve
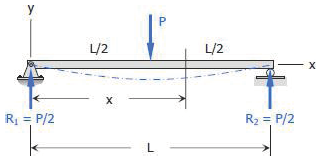
Determine the maximum deflection δ in a simply supported beam of length L carrying a concentrated load P at midspan.
E I y'' = 0.5 Px - P(x - 0.5 L)
E I y' = 0.25 P x2 - 0.5 P( x - 0.5 L )2 + C1
E I y = ( 1/12 ) P x3 - ( 1/6 ) P ( x - 0.5 L )3 + C1 x + C2
At x = 0, y = 0, therefore, C2 = 0
At x = L, y = 0
0 = ( 1/12 ) P L3 - (1/6) P ( L - 0.5 L)3 + C1 L
0 = ( 1/12 ) P L3 - ( 1/48 ) P L3 + C1 L
C1 = - ( 1/16 ) P L2
Thus,
E I y = ( 1/12 ) P x3 - (1/6) P ( x - 0.5 L)3 - (1/16) P L2 x
Maximum deflection will occur at x = ½ L (midspan)
E I ymax = ( 1/12 )P (½ L)3 - (1/6) P ( 0.5 L - 0.5 L )3 - (1/16) P L 2 ( ½ L) )
E I ymax = ( 1/96 ) P L3 - 0 - (1/32) P L3
ymax = - ( P L3 ) / ( 48 E I )
The negative sign indicates that the deflection is below the undeformed neutral axis.
Therefore,
δmax = - ( P L3 ) / ( 48 E I )
Related:
- Double Integration Method for Beam Deflections
- Beam Supported on Both Ends Uniform Loading Deflection and Stress Stress, Deflection Equation and Calculator using total applied load and line load.
- Beam Calculator Cantilevered Beam with One Load Applied at End Deflection and Stress Structural Beam Deflection, Stress, Bending Equations and calculator for a Cantilevered Beam with One Load Applied at End.
- Beam Distributed Load Deflection, Shear, Deflection and Stress Equations and Calculator Beam Deflection, Shear and Stress Equations and Calculator for a Beam supported One End, Pin Opposite End and Partial Distributed Load
- Calculator for a Beam supported One End, Pin Opposite End and Single Moment at End Applied Deflection and Stress Calc Beam Deflection, Shear and Stress Equations and Calculator for a Beam supported One End, Pin Opposite End and Single Moment at End Applied
- Stress & Deflection Deflection Equations and Calculator Beam Supported Uniform loading Applied on Cantilevered End Stress Deflection Equations and Calculator Beam Supported Uniform loading Applied on Cantilevered End
Reference:
- Dr. ZM Nizam Lecture Notes
- Shingley Machine Design, 4-3 "Deflection Due to Bending"
- Beam Deflection by Integration Lecture Presentation Paul Palazolo, University of Memphis,
- Beam Deflections Using Double integration, Steven Vukazich, San Jose University