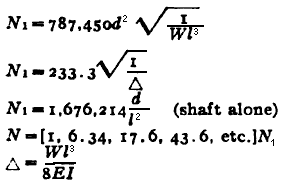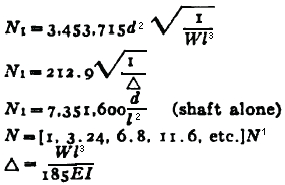Critical Speeds of Rotating Shafts with Distributed Loads - First Critical Speed

Bearings Application Knowledge Menu
Critical Speeds of Rotating Shafts or Mass Review

Critical Speeds of Rotating Shafts with Distributed Loads - First Critical Speed : When calculating critical speeds, the weight or mass of the rotating cylinder or shaft is assumed to be zero or add 1/2 to 2/3 of the rotating shaft to the load mass. These formulas assume steel shafts having a modulus of elasticity E = 29,000,000. Keep in mind that a shaft with more than one load or distributed loads may have an infinite number of critical speeds. Typically, the first critical speed is most important for your design.

Load Configuration	Equation
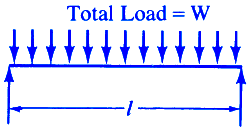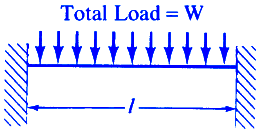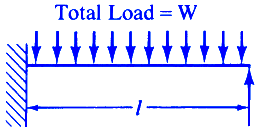	Open: Critical Speeds Rotating Shaft Supported Ends Distributed Load Calculator
	Open: Critical Speeds Rotating Shaft Fixed Ends Distributed Load Calculator
	Open: Critical Speeds Rotating Shaft Fixed End Distributed Load Calculator
	Open: Critical Speeds Rotating Shaft Fixed End Distributed Load Calculator

 

N	= critical speed, RPM
d	= diameter of shaft, (inches, mm)
W	= load applied to shaft, (lbs, Kg)
Δ	= maximum shaft deflection, (inches, mm)
l	= distance between centers of bearings, (inches, mm)
a	= distance from bearings to load, (inches, mm)
b	= distance from bearings to load, (inches, mm)

Related Resources:

• Critical Speeds of Rotating Shafts with Single Loads Equations
• Critical Speeds of Rotating Shafts Review