Beam on Elastic Foundation Case 1 Calculator and Equations

Related Resources: calculators

Beam on Elastic Foundation Case 1 Calculator and Equations

Beam Deflection and Stress Calculators with Formulas

Beam on Elastic Foundation Calculator and Equations Loading Case 1

Single load at center of Beam

Elastic Beam Loading Single Point

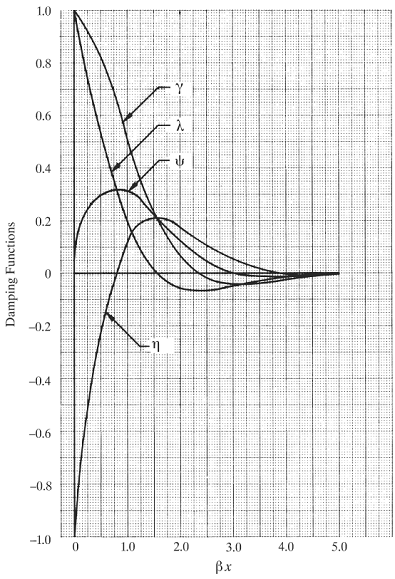
When a beam rests on one or more elastic foundations the final beam deflection and internal shears and bending moment depends on the foundation stiffness as well as the beam stiffness. To make the equation convenient the foundation constant β is available from Figure 1 and the damping functions (γ, λ, ψ, η,) are obtained from Figure 2.

Preview: Beam on Elastic Foundation Calculator and Equations Loading Case 1

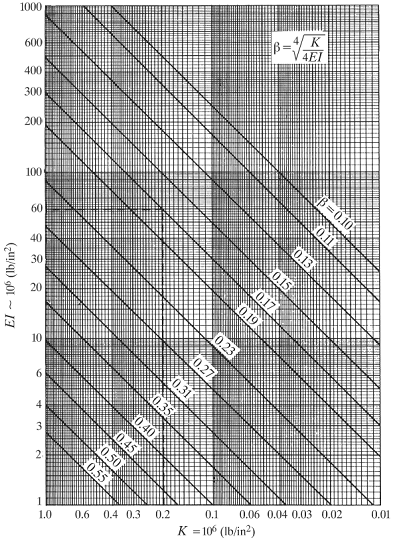
Figure. 1. Foundation Constant β

Figure 2 Damping Constants

Eq. 1
M = -P ( η ) / ( 4 β )

Eq. 2
V = -P ( λ ) / 2

Eq. 3
y = P β ( γ ) / ( 2 K )

Eq. 4
K = p / y

Eq. 5
β = [ K / ( 4 E I ) ] ^(1/4)

Eq. 6
γ = e^-βx ( cos βx + sin βx )

Eq. 7
ψ = e^-βx sin βx

Eq. 8
η = e^-βx ( sinβx - cosβx )

Eq. 9
λ = e^-βx cos βx

Declarations

P = Applied Load (lbs)
p = Load per unit length (lbs / in)
M = Moment (in-lbs)
V = Shear (lbs)
E = Modulus of Elasticity (psi)
I = Area moment of inertia (in⁴)
K = p / y = spring constant of the foundation
p = load per unit length lbs/in
y = deflection (in)
x = distance from applied force (in)
Note: The beam must extend a minimum distance x = 5 / β beyond the applied loads of moments
β = Foundation constant
ψ = damping constant (Figure 2)
η = damping constant (Figure 2)
γ = damping constant (Figure 2)
λ = damping constant (Figure 2)

Credits:

M. Hete’nyi, “Beams on Elastic Foundation”