Related Resources: calculators
Counterbalancing Several Masses Located in a Single Plane Formulas and Calculator
Engineering Materials
Tolerances, Engineering Design Limits ans Fits
Counterbalancing Several Masses Located in a Single Plane Formulas and Calculator
In all balancing problems, the product of the counterbalancing mass (or weight) and its radius are calculated; it is thus necessary to select either the mass or the radius and then calculate the other value from the product of the two quantities. Design considerations usually make this decision self-evident. The angular position of the counterbalancing mass must also be calculated.

Calculator requires a Premium membership
Preview Counterbalancing Several Masses Located in a Single Plane Calculator
Counterbalancing Several Masses Located in a Single Plane can be calculated by the following formulas:
Eq :
MB rB = [ ( ∑ M r cosθ )2 + ( ∑ M r sinθ )2 ]1/2
tanθB = - ( ∑ M r sinθ) / - ( ∑ M r cosθ ) = - y / - x
Image and Table 1
Relationship of Angle Function Signs to Quadrant in Which They Occur
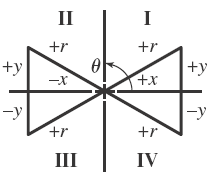
Angle θ |
||||
Trigonmetric Function |
0° to 90° |
90° to 180° |
180° to 270° |
270° to 360° |
Signs of the Functions |
||||
tan |
+y /
+x |
+y / -x |
-y / -x |
-y / +x |
sine |
+y / +r |
+y / +r |
-y / +r |
-y / +r |
cosine |
+x / +r |
-x / +r |
-x / +r |
+x / +r |
Where:
M1, M2, M3, ..., Mn = any unbalanced mass or weight, (kg, lb),
MB = counterbalancing mass or weight, (kg, lb),
r = radius to center of gravity of any unbalance mass or weitgh, (mm, in),
rB = radius to center of gravity of counterbalancing mass or weight, (mm, in),
θ = angular position of r of any unbalanced mass or weight, (degrees),
θB = angular position of rB of counterbalancing mass or weight, degrees
Reference:
Machinery's Handbook 30th edition
Related:
- Computational Dynamics
- Statics and Dynamics , Introduction
- Design Guide Natural Frequencies Straight and Curved Beams
- Natural Frequency Electric Motor Equation and Calculator
- Dynamic Resonance Review
- Simple Pendulum Natural Frequency and Period Equations and Calculator
- Angular Natural Frequency Geared System Equations and Calculator
- Angular Natural Frequency Shaft and One Mass Equations and Calculator
- Angular Natural Frequency Shaft Two Masses Equations and Calculator