Helical Spring Fatigue Loading and Factor of Safety Formulas and Calculator

Related Resources: calculators

Helical Spring Fatigue Loading and Factor of Safety Formulas and Calculator

Helical Spring Fatigue Loading and Factor of Safety Formula and Calculator

Rarely are helical springs not subjected to fatigue loading. The number of cycles may only be in hundreds or thousands, but usually they must be designed for millions and millions of cycles such that an infinite life is desired.

Helical springs may be subjected to completely reversed loading, where the mean shear stress (τ_m) is zero; however, as this type of spring is installed with a preload, the spring is usually subjected to fluctuating loading. The fluctuating loading may be compressive or tensile, but never both.

If the maximum force on the spring is denoted as (Fmax) and the minimum force is denoted as (F_min), whether they are compressive or tensile, then the mean force (F_m) and alternating force (F_a) are given by the relationships in Eqs. 1 and 2.

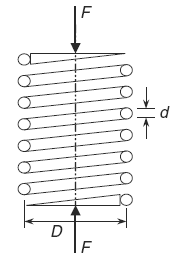
Helical Compression Spring

Preview Helical Spring Fatigue Loading and Factor of Safety Calculator

Mean spring force

Eq 1
F_m = ( F_max + F_min ) / 2

Alternating spring force

Eq. 2
F_a = ( F_max - F_min ) / 2

Stress-concentration factors are applied only to the alternating stresses the mean shear stress (τ_m) is given by:

Eq. 3
τ_m = K_s ( 8 F_m D ) / ( π d^{3 )}

where is the shear-stress correction factor

Eq. 4
K_s = 1 / ( 2 C ) + 1 = ( 1 + 2 C ) / ( 2 C )

and

C = D / d ≤ C ≤ 12

Using the Bergstrasser factor ( K_B ) in place of the shear-stress correction factor ( K_s ), the alternating shear stress ( τ_a ) is:

Eq. 5
τ_a = K_B ( 8 F_a D ) / ( π d³ )

and

K_B = ( 4C + 2 ) / ( 4C -3)

Also, the Goodman theory for fluctuating torsional loading is applicable where the factor of safety (n) for a safe design was given by:

Eq. 6
(Goodman Theory)
τ_a / S_e + τ_a / S_us = 1 / n

where the endurance limit (S_e) is calculated as usual using the Marin formula with the load type factor (k_c) equal to (0.577), and the ultimate shear stress ( S_us ) found from the relationship:

Eq. 7
S_us = ( 0.67 ) S_ut

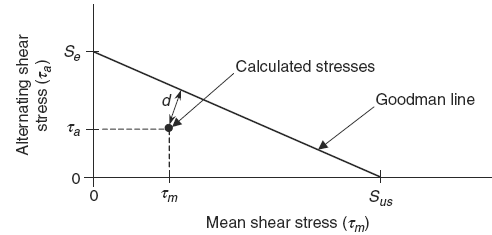
The Goodman theory given in Eq. (6) can be represented graphically as:

Figure 2

The perpendicular distance (d) to the Goodman line in Fig. 2 represents how close the factor-of-safety (n) is to the value of 1.

Once the mean shear stress (τ_m), the alternating shear stress (τ_a), the endurance limit (S_e), and the ultimate shear strength (S_us) are known, the factor-of-safety (n) for the design can be determined either mathematically using Eq. 8) or graphically using figure 2.

Factor of safety

Eq. 8
τ_a / S_e + τ_m / S_us = 1 / n

Where:

F_min = minimum force on the spring (lb, N)
F_maxmax = maximum force on the spring (lb, N)
D = spring coil mean diameter ( in, m )
d = section diameter ( in, m )
S_e = endurance limit (kpsi, MPa)
S_us = ultimate shear stress(kpsi, MPa)

Source:

Mark's Calculations for Machine Design
Thomas H. Brown, Jr.
Faculty Associate
Institute for Transportation Research and Education
NC State University
Raleigh, North Carolina