Nonuniform Diameter Change of Length Under Axial Force Formula and Calculator

Related Resources: calculators

Nonuniform Diameter Change of Length Under Axial Force Formula and Calculator

Bolt & Screw Torque Charts and Equations

Rod of Nonuniform Diameter, Change of Length Under Axial Force Formula and Calculator

When applying equal and opposite forces to the ends of a rod of nonuniform diameter, as shown in Figure 1. If the tension stress created in the rod is below the proportional limit, we can use Hooke’s law and the relationship between springs in series to compute the change in length of the rod.

Preview: Rod of Nonuniform Diameter, Change of Length Under Axial Force Calculator

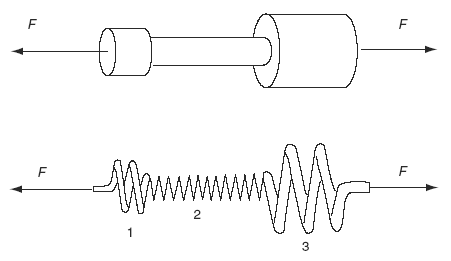
Figure 1 Rod of nonuniform diameter, loaded in tension, and equivalent spring model.

The combined change in length of the rod will be equal to the sum of the changes in each section:

Equation 1
ΔL_c = ΔL₁ + ΔL₂ + ΔL₃ + ΔL_n...

Hooke’s law tells that the change in one section will be:

Equation 2
ΔL = (F · L ) / ( E · A )

Where

ΔL = change in length (in., mm)
A = cross-sectional area (in.², mm²)
L = length of the section (in., mm)
E = modulus of elasticity (psi, GPa)
F = applied tensile force (lb, N)

Since the various sections are connected in series, they each see the same force, so we can combine Equations 1 and 2 above and write

Equation 3
ΔL_c = F [ L₁ / ( E₁ A₁ ) + L₂ / ( E₂ A₂ ) + L₃ / ( E₃ A₃ ) + L_n / ( E_n A_n ) + ... ]

the spring constant of a body is defined as

Equation 4
K = F / ΔL

Where

K = spring constant or stiffness (lb/in., N/mm)
ΔL = change in length of the body under load (in., mm) F
F = applied load (lb, N)

The spring constant of a group of bodies, connected in series, is

Equation 5
1 / K _T = 1 / K ₁ + 1 / K ₂ + 1 / K ₃ + 1 / K _n + ...

Where

K _T = combined spring constant of the group (lb/in., N/mm)
K ₁, K ₂, . . . = spring constants of individual members of the group (lb/in., N/mm)

equation for the spring constant of a body can be rewritten as

Equation 6
ΔL = ( F / K ) or ΔL = F ( 1 / K )

Comparing our equation for the spring constant for a group of bodies to the equation for the stretch or change in length of a group of bodies, we see that

Equation 7
( 1 / K _T ) = L₁ / ( E₁ A₁ ) + L₂ / ( E₂ A₂ ) + L₃ / ( E₃ A₃ ) + L_n / ( E_n A_n ) + ...

ΔL_c = F [ L₁ / ( E₁ A₁ ) + L₂ / ( E₂ A₂ ) + L₃ / ( E₃ A₃ ) + L_n / ( E_n A_n ) + ... ]

Reference:

Introduction to the Design and Behavior of Bolted Joints Non-Gasketed Joints, Forth Edition
Founding Editor
L. L. Faulkner
Columbus Division, Battelle Memorial Institute,
Department of Mechanical Engineering
The Ohio State University
Columbus, Ohio