Related Resources: calculators
Shock Loading Helical Springs Formulas and Calculator
Manufacturing Design
Spring Design and Engineering Resources
Stresses in Helical Springs Produced by Shocks Formulas and Calculator
Preview: Stresses in Helical Springs Produced by Shocks Calculator
Stresses in Helical Springs Produced by Shocks. A load suddenly applied on a spring will produce the same deflection, and, therefore, also the same unit stress, as a static load twice as great. When the load drops from a height h, the stresses are as given in the accompanying Table 1. The approximate values are applicable when the deflection is small as compared with the height h. The formulas show that the fiber stress for a given shock will be greater in a spring made from a square bar than in one made from a round bar, if the diameter of coil is the same and the side of the square bar equals the diameter of the round bar. It is, therefore, more economical to use round stock for springs which must withstand shocks, due to the fact that the deflection for the same fiber stress for a square bar spring is smaller than that for a round bar spring, the ratio being as 4 to 5. The round bar spring is therefore capable of storing more energy than a square bar spring for the same stress.
Round Bar Spring
Fiber (Unit) Stress f Produced by Weight Q Dropped a Height h on a Helical Spring
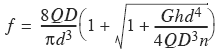
Approximate Value of f Round Bar Spring
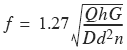
Square Bar Spring
Fiber (Unit) Stress f Produced by Weight Q Dropped a Height h on a Helical Spring
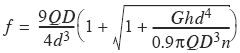
Approximate Value of f Square Bar Spring
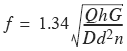
Where:
Q = Weight
G = modulus of elasticity for torsion;
d = diameter or side of bar;
D = mean diameter of spring;
n = number of coils in spring.
f = fiber unit stress
Reference: Machinerys Handbook 29th Edition
Related:
- Spring Terminology and Definitions | Engineers Edge
- Spring Design and Engineering, Formulas - Engineers Edge
- Belleville Spring Washer Equation and Calculator | Engineers Edge
- Spring Types and Materials Review | Engineers Edge
- Morrison's Spring Tables Design Reference | Engineers Edge | www.engineersedge.com