Related Resources: calculators
Stresses in Universal Knuckle Joint Formulas and Calculator
Machine Design Applications
Beam Stress and Deflection Formulas and Calculators
Stresses in Universal knuckle joint formulas and calculator
Universal joints are a mechanical form of coupling, originally known as a Cardan or Hooke's coupling, is used for connecting two shafts the axes of which are not in line with each other, but which merely intersect at a point. There are many different designs of universal joints or couplings, which are based on the principle embodied in the original design.
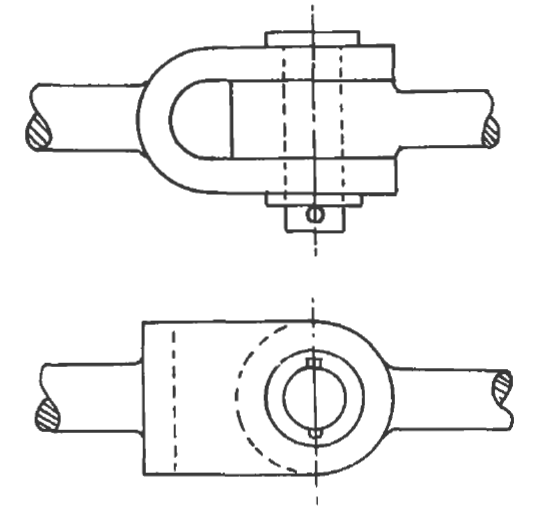
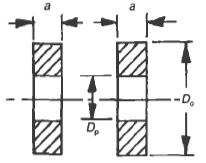
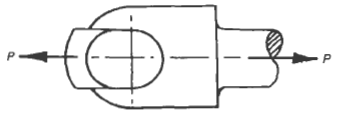
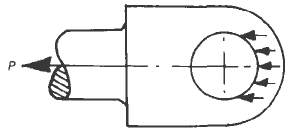
Universal Knuckle Joint
The universal knuckle joint is a good example of the application of simple stress calculations. The various stresses which may occur are given below. Failure of the universal joint may be due to any one of the stresses exceeding the material capabilities.
Preview: Stresses in Universal Knuckle Joint Calculator
Formula Symbol declarations:
P = load
σt = tensile stress
σb = bending stress
σc = crushing stress
τ = shear stress
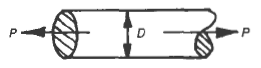
D = rod diameter
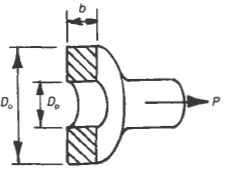
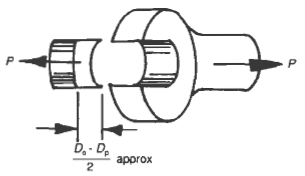
Dp = Pin diameter
Do = eye outer diameter
a = thickness of the fork
b = the thickness of the eye
(1) Tensile Stress in Shear Pin formula:
σt = 4 P / ( π D2 )
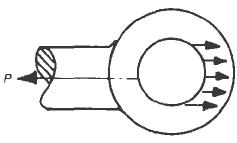
(2) Tensile Stress in Universal Joint Clevis Eye Section Formula:
σt = P / ( ( Do - Dp ) b )
(3) Shear Stress in Clevis Eye Formula:
τ = P / ( ( Do - Dp ) / b )
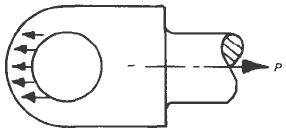
(4) Tensile Stress in Clevis Fork Section:
σt = P / ( ( Do - Dp ) / 2a )
(5) Shear Stress in Fork Section Formula:
τ = P / ( ( Do - Dp ) 2a )
(6) Crushing Stress in Universal Joint Eye Formula:
σc = P / ( b Dp )
(7) Crushing stress in fork Formula:
σc = P / ( 2 Dp a )
(8) Shear Stress in Universal Joint Pin Formula:
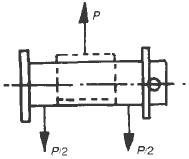
τ = 2 P / ( π Dp2 )
(9) Bending Stress in Universal Joint Pin Formula:
σb = [ 4 P ( a + b ) ] / ( π Dp3 )
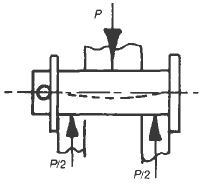
(10) Crushing Stress in Pin Due to Eye Forces Formula:
σc = P / ( b Dp )

(11) Crushing Stress in Pin Due to Fork Formula:
σc = P / ( 2 a Dp )
Related:
- Truck and Car Universal Joint Design and Engineering Equation
- Universal Joint Maximum Minimum Speed | Engineering Reference and Online Tools
Reference:
Mechanical Engineering Data Handbook, James Carvill, 2003