Related Resources: calculators
Tilting Pad Thrust Plate Bearing Design Equation and Calculator
Machine Design Applications
Bearing Engineering and Design
Tilting Pad Thrust Plate Bearing Design Equation and Calculator:
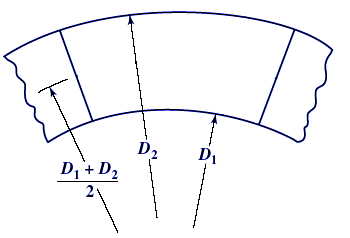
Each bearing section is wedge shaped, as shown at the right below, for the purposes of design calculation, it is considered to be a rectangle with a length b equal to the circumferential length along the pitch line of the section being considered and a width a equal to the difference in the external and internal radii,
Related:
- Flat Thrust Plate Bearing Design Formulas and Calculator
- Tapered Land Thrust Plate Bearing Design Formulas and Calculator
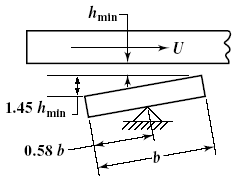
Tilting Pad Thrust Plate Bearing
Preview: Tapered Land Thrust Plate Bearing Design Calculator
|
Thrust Bearing Typical Loads
|
||
|
Surface
|
Loads
Lbs/in2 |
Max Loads
Lbs/in2 |
|
Parallel surface
|
< 75
|
< 150
|
|
Step Surface
|
200
|
500
|
|
Tapered Land Surface
|
200
|
500
|
|
Tilting Pad Surface
|
200
|
500
|
Table p
Reproduced with permission from Wilcock and Booser, Bearing Design and Applications, McGraw-Hill Book Co., Copyright © 1957.
External diameter formula:
D2 = ( ( 4 W ) / ( ( π Kg p ) + D12 )1/2
Where:
W = applied load, pounds
Kg = fraction of circumference occupied by pads; usually, 0.8
p = unit load, see Table p
Radial pad width , given in inches
a = (1/2) ( D2 - D1 )
Pitch line circumference , given in inches
B = π (D1 + D2 ) / 2
Number of bearing pads, estimated
i = ( B Kg ) / a = nearest even number
i as the nearest even number to that calculated.
Length of bearing pad given in inches
b = ( B Kg ) / i
Operating number
O = ( 1.45 x 10-7 Z2 U ) / ( 5 p b z)
Where, Z2 = viscosity of oil at outlet temperature (inlet temperature assumed temperatur rise through the bearing).
Reproduced with permission from Wilcock and Booser, Bearing Design and Applications, McGraw-Hill Book Co., Copyright © 1957.
Minimum film thickness given in mils inches - hmin = α b
α = dimensionless film thickness is found from Film Thickness Factor Chart
In general, this value should be 0.001 inch for small bearings and 0.002 inch for larger and high-speed bearings.
Coefficient of friction, f found from Coefficient of Friction Chart
Friction power loss (HP), derived from table using film thickness h
Pf = ( f W U ) / 33,000
Actual oil flow, given in gpm
Q = 0.0591 α i a b U
Δt = ( 0.0217 f p ) / ( α c )
Where:
c = specific heat of oil in Btu/gal/°F
Notation:
a = radial width of pad, inches
b = circumferential length of pad at pitch line, inches
b2 = pad step length
B = circumference of pitch circle, inches
c = specific heat of oil, Btu/gal/°F
D = diameter, inches
e = depth of step, inch
f = coefficient of friction
g = depth of 45° chamfer, inches
h = film thickness, inch
i = number of pads
J = power loss coefficient
K = film thickness factor
Kg = fraction of circumference occupied by the pads; usually, 0.8
l = length of chamfer, inches
M = horsepower per square inch
N = revolutions per minute
O = operating number
p = bearing unit load, psi
ps = oil-supply pressure, psi
Pf = friction horsepower
Q = total flow, gpm
Qc = required flow per chamfer, gpm
Qoc = uncorrected required flow per chamfer, gpm
QF = film flow, gpm
s = oil-groove width
∆t = temperature rise, °F
U = velocity, feet per minute
V = effective width-to-length ratio for one pad
W = applied load, pounds
Yg = oil-flow factor
Yl = leakage factor
YS = shape factor
Z = viscosity, centipoises
α = dimensionless film-thickness factor
δ = taper
ξ = kinetic energy correction factor
References:
- Machinery's Handbook, 29th Edition
- Understanding Journal Bearings, Malcolm E. Leader, P.E. Applied Machinery Dynamics Co.
- Theory and Practice of Lubrication for Engineers by Dudley D. Fuller, Wiley and Sons, 1984, ISBN 0- 471-04703-1
- Bearing Design and Application by Donald F. Wilcock and E. Richard Booser, McGraw Hill, 1957, 195, LC number 56-9641