Ideal Pinned Column Buckling Calculation and Equation

Ideal Pinned Column Buckling Equation and Calculation, Euler's Formula

For the ideal pinned column shown in below, the critical buckling load can be calculated using Euler's formula:

Euler's Formula

Open: Ideal Pinned Column Buckling Calculator

Where:

E = Modulus of elasticity of the material
I = Minimum moment of inertia
L = Unsupported length of the column (see picture below)

Note that regardless of the end condition, the critical load depends not on the material strength, but rather the flexural rigidity, EI. Buckling resistance can be increased by increasing the moment of inertia.

When a column buckles, it maintains its deflected shape after the application of the critical load. In most applications, the critical load is usually regarded as the maximum load sustainable by the column. Theoretically, any buckling mode is possible, but the column will ordinarily deflect into the first mode. A column will buckle when the load P reaches a critical level, called the critical load, P_cr.

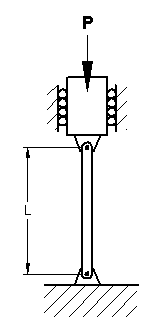
Ideal Pinned Column (Pinned - Pinned)

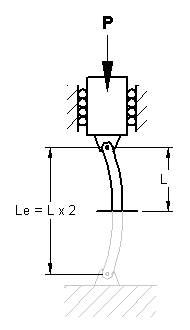
For columns with different types of support, Euler's formula may still be used if the distance L is replaced with the distance between the zero moment points. See "Effective Length Constant Table" below.

This length is called the effective length Le and is illustrated in below. Thus the critical load equation becomes:

critical load equation

slenderness ratio

The slenderness ratio is an important parameter in the classification of compression members, and is represented by the equation:

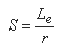
slenderness ratio

Where:

r = Radius of gyration
I = Moment of inertia
A = Area cross section

If the slenderness ratio > (greater than) critical slenderness ratio, then the column is treated as a long column and the Euler buckling formula is applicable.

Euler buckling formula

If slenderness ratio is < (less than) the critical slenderness ratio, the column is treated as a short column.

In short columns, failure may occur by compression without significant buckling and at stresses exceeding the proportional limit. For this condition, Johnson's formula is applicable:

proportional limit

For columns that fail subsequent to the onset of inelastic behavior, the constant of proportionality must be used rather than the modulus of elasticity (Engesser formula). The constant of proportionality, Et, is the slope of the stress-strain diagram beyond the proportional limit, termed the tangent modulus. Note within the linearly elastic range, E = Et.

Effective Length Constant Table
Column Boundary	Effective Length Constant ** C = L^e / L
Free - Free	1.0
Fixed - Free	2.0
Fixed - Pinned	.707
Fixed - Guided	1.0
Fixed - Fixed	.5
Guide - Free	2.0
Guided - Pinned	2.0
Guided - Guided	1.0
Pinned - Free	1.0
Pinned - Pinned	1.0

** In practice, the Effective Length Constant is increased by a factor approximately 10 - 20 %

Open: Ideal Pinned Column Buckling Calculator