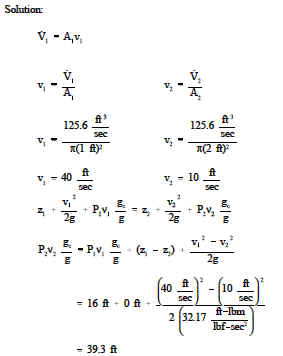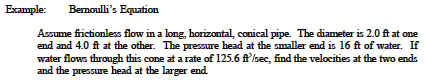Energy Conversions in Fluid Systems Bernoullis Equation

Fluid Flow Table of Contents
Hydraulic and Pneumatic Knowledge

Energy Conversions in Fluid Systems Bernoullis Equation

Bernoullis equation makes it easy to examine how energy transfers take place among elevation head, velocity head, and pressure head. It is possible to examine individual components of piping systems and determine what fluid properties are varying and how the energy balance is affected.

If a pipe containing an ideal fluid undergoes a gradual expansion in diameter, the continuity equation tells us that as the diameter and flow area get bigger, the flow velocity must decrease to maintain the same mass flow rate. Since the outlet velocity is less than the inlet velocity, the velocity head of the flow must decrease from the inlet to the outlet. If the pipe lies horizontal, there is no change in elevation head; therefore, the decrease in velocity head must be compensated for by an increase in pressure head. Since we are considering an ideal fluid that is incompressible, the specific volume of the fluid will not change. The only way that the pressure head for an incompressible fluid can increase is for the pressure to increase. So the Bernoulli equation indicates that a decrease in flow velocity in a horizontal pipe will result in an increase in pressure.

If a constant diameter pipe containing an ideal fluid undergoes a decrease in elevation, the same net effect results, but for different reasons. In this case the flow velocity and the velocity head must be constant to satisfy the mass continuity equation.

So the decrease in elevation head can only be compensated for by an increase in pressure head. Again, the fluid is incompressible so the increase in pressure head must result in an increase in pressure.

Although the Bernoulli equation has several restrictions placed upon it, there are many physical fluid problems to which it is applied. As in the case of the conservation of mass, the Bernoulli equation may be applied to problems in which more than one flow may enter or leave the system at the same time. Of particular note is the fact that series and parallel piping system problems are solved using the Bernoulli equation.