Conduction - Cylindrical Coordinates - Heat Transfer

Heat Transfer Engineering | Thermodynamics

Heat transfer across a rectangular solid is the most direct application of Fouriers law. Heat transfer across a pipe or heat exchanger tube wall is more complicated to evaluate. Across a cylindrical wall, the heat transfer surface area is continually increasing or decreasing. Figure 3 is a cross-sectional view of a pipe constructed of a homogeneous material.

The surface area (A) for transferring heat through the pipe (neglecting the pipe ends) is directly proportional to the radius (r) of the pipe and the length (L) of the pipe.

A = 2 π r L

As the radius increases from the inner wall to the outer wall, the heat transfer area increases. The development of an equation evaluating heat transfer through an object with cylindrical geometry begins with Fouriers law Equation 2-5.

From the discussion above, it is seen that no simple expression for area is accurate. Neither thearea of the inner surface nor the area of the outer surface alone can be used in the equation. For a problem involving cylindrical geometry, it is necessary to define a log mean cross-sectional area (A_lm).

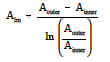
Substituting the expression 2 p>rL for area in Equation 2-7 allows the log mean area to be calculated from the inner and outer radius without first calculating the inner and outer area.

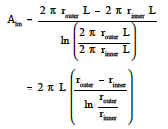(Equation 2-7)

This expression for log mean area can be inserted into Equation 2-5, allowing us to calculate the heat transfer rate for cylindrical geometries.

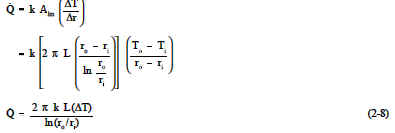
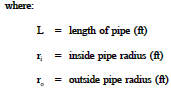
Example:

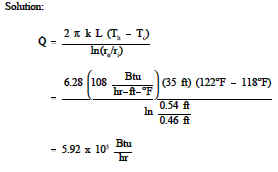
A stainless steel pipe with a length of 35 ft has an inner diameter of 0.92 ft and an outer diameter of 1.08 ft. The temperature of the inner surface of the pipe is 122^oF and the temperature of the outer surface is 118 ^oF. The thermal conductivity of the stainless steel is 108 Btu/hr-ft->^oF.

Calculate the heat transfer rate through the pipe. 

Calculate the heat flux at the outer surface of the pipe.

Example:

A 10 ft length of pipe with an inner radius of 1 in and an outer radius of 1.25 in has an outer surface temperature of 250F. The heat transfer rate is 30,000 Btu/hr. Find the interior surface temperature. Assume k = 25 Btu/hr-ft-F.

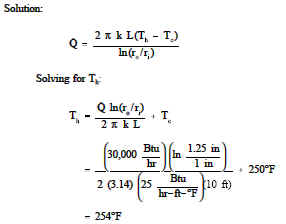
The evaluation of heat transfer through a cylindrical wall can be extended to include a composite body composed of several concentric, cylindrical layers, as shown in Figure 4.

Example:

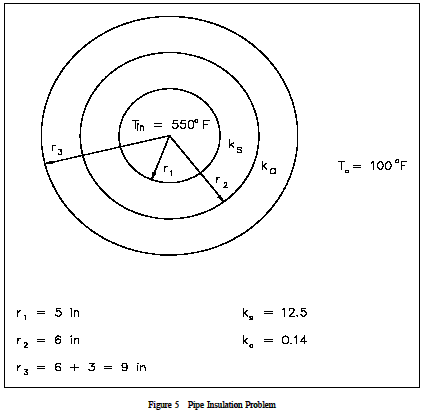
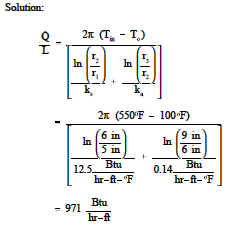
A thick-walled nuclear coolant pipe (k_q= 12.5 Btu/hr-ft-F) with 10 in. inside diameter (ID) and 12 in. outside diameter (OD) is covered with a 3 in. layer of asbestos insulation (k_a= 0.14 Btu/hr-ft-_oF) as shown in Figure 5. If the inside wall temperature of the pipe is maintained at 550F, calculate the heat loss per foot of length. The outside temperature is 100F.