Related Resources: heat transfer
Heat Loss Insulated Pipe Equation and Calculator
Heat Transfer Engineering
Thermodynamics
Engineering Physics
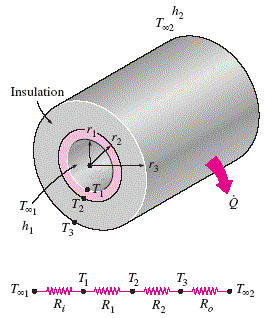
Heat Loss through a Insulated Pipe Equation and Calculator:
Assumptions:
1 Heat transfer is steady since there is no indication of any change with time.
2 Heat transfer is one-dimensional since there is thermal symmetry about the centerline and no variation in the axial direction.
3 Thermal conductivities are constant.
4 The thermal contact resistance at the interface is negligible.
ALL calculators require a Premium Membership
Preview: Heat Loss Through a Insulated Pipe Calculator
Areas of the surfaces exposed to convection:
![]()
Individual thermal resistances:
![]()
![]()
![]()
![]()
All resistances are in series, the total resistance is:
![]()
Steady rate of heat loss:
![]()
The heat loss for a given pipe length can be determined by multiplying the above quantity by the pipe length L.
Temperature drops across the pipe and the insulation
![]()
![]()
Where:
Q = Heat Steady State Transfer (W)
A = Area (m2)
L = Length (m)
rn = Radius (m)
k = Thermal Conductivity (W/m · °C)
T∞n = Temperature (°C)
Tn = Temperature (°C)
hn = Heat Transfer Coefficient (W/m2 · °C)
Rconv, n = Thermal Resistance (°C/W)
Rinsulation1 = Thermal Resistance Glass (°C/W)