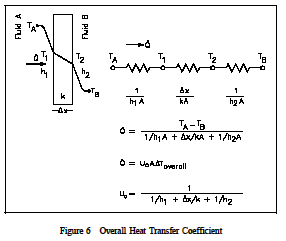
Combined Overall Heat Transfer Coefficient Equation

Heat Transfer Engineering | Thermodynamics

Many of the heat transfer processes encountered in nuclear facilities involve a combination of both conduction and convection. For example, heat transfer in a steam generator involves convection from the bulk of the reactor coolant to the steam generator inner tube surface, conduction through the tube wall, and convection from the outer tube surface to the secondary side fluid. In cases of combined heat transfer for a heat exchanger, there are two values for h. There is the convective heat transfer coefficient (h) for the fluid film inside the tubes and a convective heat transfer coefficient for the fluid film outside the tubes. The thermal conductivity (k) and thickness (D_x) of the tube wall must also be accounted for. An additional term (U_o), called the overall heat transfer coefficient, must be used instead. It is common practice to relate the total rate of heat transfer ( ) to the cross-sectional area for heat transfer (A_o) and the overall heat ˙Q transfer coefficient (U_o). The relationship of the overall heat transfer coefficient to the individual conduction and convection terms is shown in Figure 6.

Overall Heat Transfer Coefficient

Recalling Equation 2-3:

˙Q =U_oA_oDTo

where U_ois defined in Figure 6.

An example of this concept applied to cylindrical geometry is illustrated by Figure 7, which shows a typical combined heat transfer situation.

Using the figure representing flow in a pipe, heat transfer by convection occurs between temperatures T₁ and T₂; heat transfer by conduction occurs between temperatures T₂and T₃; and heat transfer occurs by convection between temperatures T₃and T₄. Thus, there are three processes involved. Each has an associated heat transfer coefficient, cross-sectional area for heat transfer, and temperature difference. The basic relationships for these three processes can be expressed using Equations 2-5 and 2-9.

˙Q =h₁A₁(T_o1-₂)

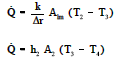
heat transfer by convection

DT_ocan be expressed as the sum of the DT of the three individual processes.

If the basic relationship for each process is solved for its associated temperature difference and substituted into the expression for _D T_o above, the following relationship results.

associated temperature difference

This relationship can be modified by selecting a reference cross-sectional area A_o.

Solving for Q results in an equation in the form

. Solving for Q results in an equation in the form

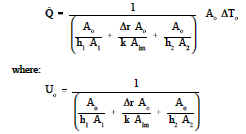
Equation 2-10 for the overall heat transfer coefficient in cylindrical geometry is relatively difficult to work with. The equation can be simplified without losing much accuracy if the tube that is being analyzed is thin-walled, that is the tube wall thickness is small compared to the tube diameter. For a thin-walled tube, the inner surface area (A₁), outer surface area (A₂), and log mean surface area (A_1m), are all very close to being equal. Assuming that A₁, A₂2, and A_1m are equal to each other and also equal to A_o allows us to cancel out all the area terms in the denominator of Equation 2-11.

This results in a much simpler expression that is similar to the one developed for a flat plate heat exchanger in Figure 6.

flat plate heat exchanger

The convection heat transfer process is strongly dependent upon the properties of the fluid being considered. Correspondingly, the convective heat transfer coefficient (h), the overall coefficient (U_o), and the other fluid properties may vary substantially for the fluid if it experiences a large temperature change during its path through the convective heat transfer device. This is especially true if the fluids properties are strongly temperature dependent. Under such circumstances, the temperature at which the properties are "looked-up" must be some type of average value, rather than using either the inlet or outlet temperature value. For internal flow, the bulk or average value of temperature is obtained analytically through the use of conservation of energy. For external flow, an average film temperature is normally calculated, which is an average of the free stream temperature and the solid surface temperature. In any case, an average value of temperature is used to obtain the fluid properties to be used in the heat transfer problem. The following example shows the use of such principles by solving a convective heat transfer problem in which the bulk temperature is calculated.