Related Resources: Wheatstone bridge

Wheatstone Bridge Analysis and Calculator

Instrumentation & Electronics

Wheatstone Bridge Analysis and Calculator

In the figure, Rx is the unknown resistance to be measured; R₁, R₂ and R₃ are resistors of known resistance and the resistance of R₂ is adjustable. If the ratio of the two resistances in the known leg (R₂/R₁) is equal to the ratio of the two in the unknown leg (R_x/R₃), then the voltage between the two midpoints (B and D) will be zero and no current will flow through the galvanometer e_o. If the bridge is unbalanced, the direction of the current indicates whether R₂ is too high or too low. R₂ is varied until there is no current through the galvanometer, which then reads zero.

Detecting zero current with a galvanometer can be done to extremely high accuracy. Therefore, if R₁, R₂ and R₃  are known to high precision, then R_x can be measured to high precision. Very small changes in R_x disrupt the balance and are readily detected.

At the point of balance, the ratio of

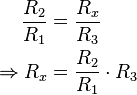
Alternatively, if R₁, R₂, and R₃ are known, but R₂ is not adjustable, the voltage difference across or current flow through the meter can be used to calculate the value of R_x, using Kirchhoff's circuit laws (also known as Kirchhoff's rules). This setup is frequently used in strain gauge and resistance thermometer measurements, as it is usually faster to read a voltage level off a meter than to adjust a resistance to zero the voltage.

Wheatstone Bridge Circuit Analysis
Finding Voltage of Meter
Cicuit Properties
Gage 1:		Resistance		Ω	Strain		με
Gage 2:		Resistance		Ω	Strain		με
Gage 3:		Resistance		Ω	Strain		με
Gage 4:		Resistance		Ω	Strain		με
	Gage Factor
	Voltage (ei)			V
Circuit Calculations
Current i1,2		=		Amps	Found using Ohm's Law: i=Vin/(R1+ΔR1+R2+ΔR2)
Current i3,4		=		Amps	Found using Ohm's Law: i=Vin/(R3+ΔR3+R4+ΔR4)
Voltage 2		=		Volts	Found using Ohm's Law: V2=i1,2*(R2+ΔR2)
Voltage 4		=		Volts	Found using Ohm's Law: V4=i3,4*(R4+ΔR4)
Voltage Meter (eo)		=		Volts	Difference between V2 and V4
Analysis
Actual Strain Values			ε1
			ε2
			ε3
			ε4
Chance in Resistance			ΔR1			Ω
			ΔR2			Ω
			ΔR3			Ω
			ΔR4			Ω