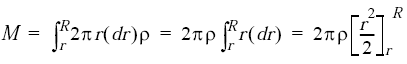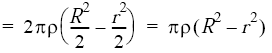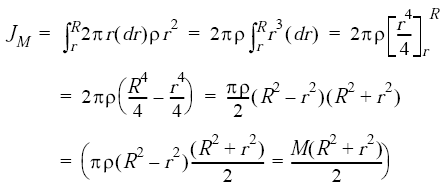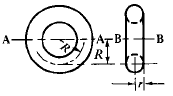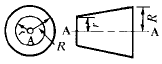Polar Mass Moment of Inertia About Axis A-A and B-B of Common Shapes

Polar Mass Moment of Inertia, Common Shape Equations and Calculator

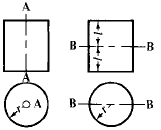
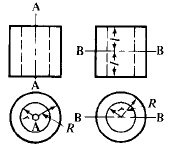
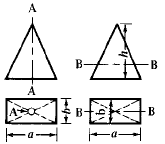
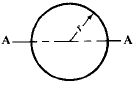
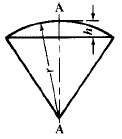
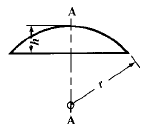
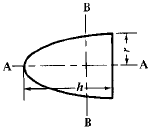
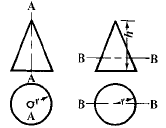
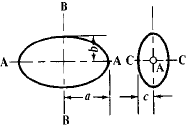
Engineering Metals and Materials Table of Contents
Engineering Calculators
Engineering Analysis Menu

Polar Mass Moment of Inertia About Axis A-A, Axis B-B and Axis C-C.

Mass Moments of Inertia, J_M. formulas for mass moment of inertia of various solids are given below.

Example, Polar Mass Moment of Inertia of a Hollow Circular Section:

A strip of width dr on a hollow circular section, whose inner radius is r and outer radius is R.

The mass of the strip = 2πrdrρ, where ρ is the density of material. In order to get the mass of an individual section, integrate the mass of the strip from r to R.

Polar Mass Moment of Inertia of a Hollow Circular Section

The 2nd moment of the strip about the AA axis = 2πrdrρr². To find the polar moment of inertia about the AA axis, integrate the 2nd moment from r to R.

2nd moment of the strip

Note: In some many engineering examples the symbol I denotes the polar moment of inertia of masses; J_M is used here to avoid confusion with moments of inertia of plane areas.

Where:

J_M = Polar Mass Moment of Inertia (in-lbs-sec^2,Kg-m-sec²)
h, b, l, L = Distance (in, m)
M = Mass = ρL = (lb - sec² / in, N - Sec² / m )
ρ = Density

All calculators require a java enabled browser and a Premium Membership Account.

Section	Mass Polar Moment of Inertia Axis A-A J_M	Mass Polar Moment of Inertia Axis B-B J_M
Mass Polar Moment of Inertia Prism ^Open Polar Mass Moment of Inertia Prism Calculator
Mass Polar Moment of Inertia Cylinder ^Open Polar Mass Moment of Inertia Cylinder Calculator
Mass Polar Moment of Inertia Hollow Cylinder ^Open Polar Mass Moment of Inertia Hollow Cylinder Calculator
Mass Polar Moment of Inertia Pyramid, Rectangular Base ^Open Polar Mass Moment of Inertia Pyramid, Rectangular Base Calculator		Through the center of gravity
Mass Polar Moment of Inertia Sphere ^Open Polar Mass Moment of Inertia Sphere Calculator
Mass Polar Moment of Inertia Spherical Sector ^Open Polar Mass Moment of Inertia Spherical Sector Calculator		-
Mass Polar Moment of Inertia Spherical Segment ^Open Polar Mass Moment of Inertia Spherical Segment Calculator		-
Mass Polar Moment of Inertia Torus ^Open Polar Mass Moment of Inertia Torus Calculator
Mass Polar Moment of Inertia Paraboloid ^Open Polar Mass Moment of Inertia Parabolid Calculator		Through the center of gravity
Mass Polar Moment of Inertia Cone ^Open Polar Mass Moment of Inertia Cone Calculator		Through the center of gravity
Mass Polar Moment of Inertia Frustum of Cone ^Open Polar Mass Moment of Inertia Frustum of Cone Calculator		-

Section	Mass Polar Moment of Inertia Axis A-A J_M	Mass Polar Moment of Inertia Axis B-B J_M	Mass Polar Moment of Inertia Axis C-C J_M
Mass Polar Moment of Inertia Ellipsoid ^Open Polar Mass Moment of Inertia Ellipsoid Calculator

Link to this Webpage:

Copy Text to clipboard