Related Resources: vibration

Beam Fixed End and Cantilevered Angular Natural Frequency Equations and Calculator

Machine Design and Engineering

Beam Fixed End and Cantilevered Angular Natural Frequency Equations and Calculator

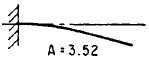l = 1

 

 

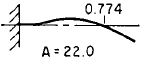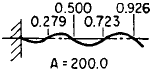ω_n = A [ ( E I ) / ( ( L l )⁴ µ ] ^(1/2)

Where:

Nodes are indiated in images as a proportion of length l measured from the the left,
L = Total Length of Beam (in),
l = Length Proportion at Node Location of Beam indicated in images (in),
I = Area Moment of Inertia of Beam Cross Section (in⁴)
E = Young's Modulus ( lb / in² ),
µ = Mass per Unit Length of Beam ( lb-sec²/in² ),
A = Coefficient for given nodes from image table above,
ω_n = Angular Natural Frequency ( rad / sec )

Related and Useful Links:

• AISC Steel Construction Shapes Properties Viewer
• Young's Modulus on Common Engineering Materials

Reference Harris, Shock and Vibration Handbook