Related Resources: vibration

Natural Frequency Three Masses Equations and Calculator

Machine Design and Engineering

Angular Natural Frequency Three Masses Axial Equations and Calculator
Mass Spring Systems in Translation Equation and Calculator

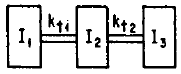
ω_n = { (1/2) [ B ± ( B² - (( 4 k_t1k_t2 ) / ( I₁ I₂ I₃ )) ( I₁ + I₂ + I₃) ) ^(1/2) ] }^(1/2)

or

ω_n+ = { (1/2) [ B + ( B² - (( 4 k_t1k_t2 ) / ( I₁ I₂ I₃ )) ( I₁ + I₂ + I₃) ) ^(1/2) ] }^(1/2)

ω_n- = { (1/2) [ B - ( B² - (( 4 k_t1k_t2 ) / ( I₁ I₂ I₃ )) ( I₁ + I₂ + I₃) ) ^(1/2) ] }^(1/2)

and

B = k_t1/ I₁ + k_t2/ I₃ + ( k_t1 + k_t2 ) / I₂

Where:

k_{t1, t2} = Torsional Stiffness of Shaft ( lb-in/rad )
I_{1, 2} = Rotary Mass Moment of Inertia of Mass ( lb-in-sec² )
ω_{n+, n-} = Angular Natural Frequency ( rad/sec )

Reference Harris, Shock and Vibration Handbook