Related Resources: vibration

Undamped Mass Spring Natural Frequency Equations and Calculator

Machine Design and Engineering

Angular Natural Frequency Undamped Mass Spring System Equations and Calculator

Mass Spring Systems in Translation Equation and Calculator

The simplest possible vibratory system is shown below; it consists of a mass m attached by means of a spring k to an immovable support.The mass is constrained to translational motion in the direction of the vertical axis so that its change of position from an initial reference is described fully by the value of a single quantity. For this reason it is called a single degree-of freedom system. If the mass m is displaced from its equilibrium position and then allowed to vibrate free from further external forces, it is said to have free vibration. The vibration also may be forced; i.e., a continuing force acts upon the mass or the foundation experiences a continuing motion.

 

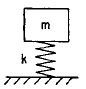
ω_n = ( k / m )^1/2

Where:

k = Spring Stiffness (lb/in)
m = Mass ( lb-sec² / in )
ω_n = Angular Natural Frequency (rad/sec)