Beam Stress Deflection Equations Calculator for Beam Fixed at One End, Supported at the Other, Load at any Point

Structural Beam Deflection, Stress, Bending Equations and calculator for a Beam Fixed at One End, Supported at the Other, Load at any Point.

Calculator for Fixed at One End, Supported at the Other, Load at any Point

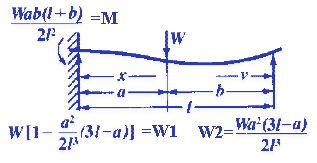
Beam Lengths m & n
(Used in many equations)

m = (l + a)(l + b) +al
n = al(l + b)

Beam Loads and Moment at Reaction points as Defined

Beam Section Modulus Z

Z = I/z

I = Moment of inertia Cross Section : Units in⁴ (m⁴)
z = Distance neutral axis to extreme fiber: Units in (m)

General Beam Stress at any Given Point

Beam Stress Between point of fixture and load

Beam Stress Between support and load

Beam Stress at Critical Locations/Points

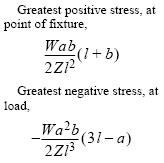
Stress at Critical Locations/Points

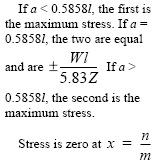
Maximum Stress

Beam Deflection at any Point

Beam Deflection Between point of fixture and load

Beam Deflection Between support and load

Beam Critical Deflections

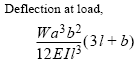
Critical Deflection Calculator

Deflection at Load Equation

Where:

E =	Modulus of Elasticity	psi	(N/mm²⁾
I =	Moment of Inertia	in⁴	(mm⁴⁾
W =	Load	lbs	(N)
s =	Beam Stress at the cross-section being evaluated	Lbs/in²	(N/mm²)
y =	Beam Deflection	inches	(mm)
x =	Some distance as indicated	inches	(mm)
u =	Some distance as indicated	inches	(mm)
a, b, c & d =	Some distance as indicated	inches	(mm)
L =	Some distance as indicated	inches	(mm)
m, n =	Some calculated distance as indicated	inches	(mm)
l =	Some distance as indicated	inches	(mm)
Z =	section modulus of the cross-section of the beam = I/z	in³	(mm³⁾
z =	distance from neutral axis to extreme fiber (edge)	inches	(mm)

Please note letter "l" (lower case "L") is different than "I" (Moment of Inertia).
Deflections apply only to constant cross sections along entire length.

References: