Related Resources: calculators

Curved T-Section Stress Formulas and Calculator

Beam Deflection and Stress Calculators with Formulas

Curved T-Section Stress Formulas and Calculator With Moment Loading Applied

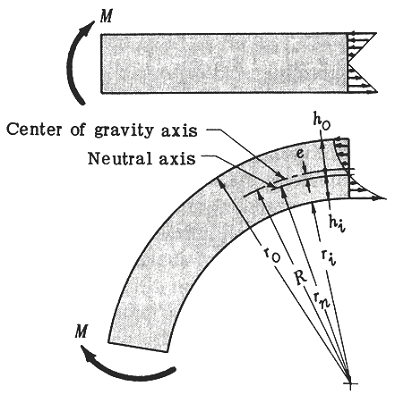
Considerable care must be taken in the arithmetic. The distance "e" from the center of gravity axis to the neutral axis is usually small. A numerical variation in the calculation of "e" can cause a large percentage change in the final results.

Preview Curved T Section Beam Stress Calculator

Figure 1.0

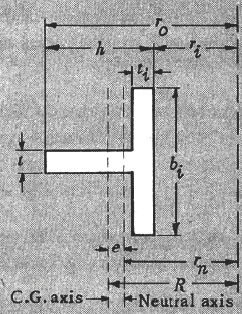
Figure 2.0 T-Section Beam Dimensions

Stress Distribution due to bending is given by:

Equation 1
s = M · γ / ( A · e · ( r_n - y ) )

Where:

s = the bending stress, psi
M = bending moment with respect to the centroical axis, in-lb
y = distance from the neutral axis to the point in question, inches (positive for distances toward the center of curvature, negative for distances away from the center of curvature)
A = the area of the section, in²
e = distance from the center of gravity axis to the neutral axis, inches
r_n = radius of curvature of the neutral axis, inches
value of e used for base log = 2.7182818

Bending Stress at the Inside Fiber is given by:

Equation 2
s = ( M · h_i ) / ( A · e · r_i )

Where:

h_i = distance from the center neutral axis to the inside fiber, inches ( h_i = r_n - r_i )
r_i = radius of curvature on the inside fiber, inches

Bending Stress at the Outside Fiber is given by:

Equation 3
s = ( M · h_o ) / ( A · e · r_i )

Where:

h_o = distance from the center neutral axis to the inside fiber, inches ( h_o = r_o - r_i )
r_o = radius of curvature on the outside fiber, inches
A = b_i t_i + (h - t_i ) t

T Section Beam Stuctural Shape

r_n = [ ( b_i - t ) t_i + th ] / [ ( b_i - t ) log_e ( ( r_i + t_i ) / r_i ) + t log_e ( r_o / t_o )]

e = R - r_n

R = r_i + [ (1/2) h² t + (1/2) t_i² ( b_i - t ) ] / [ ht + ( b_i - t ) t_i ]

Where:

b_i = T Section beam flange width, in
t_i = flange web thickness, in
t_i = flange web thickness, in
h = width, in

Related:

• Curved I-Beam Section Stress Formulas and Calculator
• Curved Circular Beam Stress Formulas and Calculator
• Curved Rectangular Beam Stress Formulas and Calculator
• Curved Beam Stress Deflection Design Spreadsheet Calculator
• Strength of Materials Basics and Equations | Mechanics of Materials
• Civil Engineering Design Knowledge
• Double Integration Method for Beam Deflections
• Double Integration Method Example 5 Proof Pinned Supported Beam
• Bending, Deflection and Stress Equations Calculator for Beam Fixed at One End, Supported at the Other, Load at Center

References:

• McGraw Hill Machine Design (1968)