Interference Pressure Mechanical Tolerances Variability Equations and Calculator

Related Resources: calculators

Interference Pressure Mechanical Tolerances Variability Equations and Calculator

Engineering, Manufacturing Tolerance Limits Fits Charts

Interference Pressure Mechanical Tolerances Variability Equations and Calculator.

Mechanical Tolerances and Fit Terminology:

When the radial interference (δ) and interface radius (R) is known, the interface pressure (p) can be calculated using equations provided on Thick Wall Cylinder Press or Shrink Fits Equations and Calculator ( Eq. 5) depending on whether the collar and shaft are made of the same material, and depending on whether the shaft is solid or hollow.

Preview: Interference Pressure from Industry Mechanical Tolerances Calculator

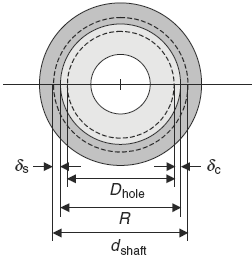
Clearance or Interference from Mechanical Tolerance Standards
Figure 1

The radial interference (δ) and the interface radius (R) are actually determined from interference fits established by ANSI (American National Standards Institute) standards. There are ANSI standards for both the U.S. customary and metric systems of units.

As the interference (δ) is associated with the changes in the radial dimensions, it can be expressed in terms of the outside diameter d_shaft of the shaft and the inside diameter D_hole of the collar given as:

Eq 1
δ = 0.5 ( d_shaft - D_hole ) = δ_c + | δ_s |

By convention, uppercase letters are used for the dimensions of the hole in the collar, whereas lowercase letters are used for the dimensions of the shaft. Also, the radial increase (δ_c) is always positive and the radial decrease (δ_s ) is always negative, which is why the
absolute value of (δ_s ) is added to (δ_c).

Fit Standards. For either the U.S. customary or metric systems of units, the web page General Mechanical Tolerance Table Charts for Standard Shaft Hole Fits contains the standards for press or shrink fits. To summarize, fits are separated into five categories:

1. Loose running and sliding fits
2. Locational clearance fits
3. Locational transition fits
4. Locational interference fits
5. Force or drive and shrink fits

Only for the fifth category, force or drive and shrink fits, does a significant interface pressure (p) develop between the shaft and collar assembly depending on the materials of the shaft and collar, and whether the shaft is hollow or solid. Note that if the interface pressure (p) exceeds the yield stress of either
the collar or the shaft, plastic deformation takes place and the stresses are different than the interface pressure calculated.

When using specific fit standards, whether U.S. customary or metric, the radial interference (δ) given by Eq. 1 needs to be separated into two different calculations. There needs to be a calculation of the maximum radial interference (δmax) to be expected that is given by:

Eq. 2
δ_max = 0.5 ( d_{max shaft} - D_{min hole} )

where (d_{max shaft}) is the maximum diameter of the shaft and (D_{min hole}) is the minimum diameter of the hole in the collar. There should also be a calculation of the minimum radial interference (δ_min) to be expected and given by:

Eq. 3
δ_min = 0.5 ( d_{min shaft} - D_{max hole} )

where (d_{min shaft}) is the minimum diameter of the shaft and D_{max hole} ) is the maximum diameter of the hole in the collar. Many times the minimum radial interference (δ_min) is zero, so the interface pressure (p) will also be zero.

The interface pressure exerted by the interference

Eq. 4
p_max = ( E δ_max ) / ( 2 R ) [ 1 - ( R / r_o ) ² ]

p_min = ( E δ_min ) / ( 2 R ) [ 1 - ( R / r_o ) ² ]

Source:

Marks' Calculations for Machine Design,
Thomas H. Brown, Jr. Ph.D., PE
Faculty Associate
Institute for Transportation Research and Education
NC State University
Raleigh, North Carolina