Centrifugal Force Equations and Calculator

Centrifugal Force - When a body of mass rotates about an axis it exerts an outward radial force called centrifugal force upon the axis or any arm or cord from the axis that restrains it from moving in a straight (tangential) line.

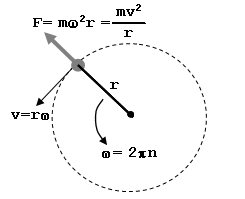
Centrifugal Force

In the following. equations:

F = Wv² / gR
F = mv² / R

Where:

F = centrifugal force in lbs or kg
m = Mass in lbs or kg
W = weight or mass of revolving body in lbs or kg
v = velocity at radius R on body in ft/sec or m/sec
g = acceleration due to gravity = 32.16 ft/ sec/sec
R = perpendicular distance in feet or meters from axis of rotation to center of mass, or for practical use, to center of gravity of revolving body

If n = number of revolutions per second

F = 1227WRn²

W = FRg / v²
W = 2933F / Rn²

v = [FRg / W]^1/2

R = Wv² / Fg
R = 2933F / Wn²

n = [ 2933 F / WR ] ^1/2

Where:

F = centrifugal force in pounds
W = weight of revolving body in pounds
v = velocity at radius R on body in feet per second
n = number of revolutions per minute
g = acceleration due to gravity = 32.16 feet per second per second
R = perpendicular distance in feet from axis of rotation to center of mass, or for practical use, to center of gravity of revolving body

Note: If a body rotates about its own center of mass, R equals zero and v equals zero. This means that the resultant of the centrifugal forces of all the elements of the body is equal to zero or, in other words, no centrifugal force is exerted on the axis of rotation. The centrifugal force of any part or element of such a body is found by the equations given below, where R is the radius to the center of gravity of the part or element. In a flywheel rim, R is the mean radius of the rim because it is the radius to the center of gravity of a thin radial section.

For SI Units:

F = Mv² / R
F = [ Mn²(2πR²) ] / (60² R)

Where:

F = centrifugal force in Newtons
M = Mass of revolving body in kilgrams
v = velocity at radius R on body in meters second
n = revolutions per second
R = perpendicular distance in meters from axis of rotation to center of mass.

Centripetal Acceleration Equations and Calculator

Link to this Webpage:

Copy Text to clipboard

Centrifugal Force
Input
Mass =
Velocity =
Radius =
Results
Force =