Related Resources: beam bending

Double Integration Method Example 5 Proof Pinned Supported Beam

Beams Deflection and Stress Formulas and Calculators
Engineering Mathematics

Double Integration Method Example 5 Proof Pinned Supported Beam of Length L with Single Cantilevered Load.

The Double Integration Method, also known as Macaulay’s Method is a powerful tool in solving deflection and slope of a beam at any point because we will be able to get the equation of the elastic curve.

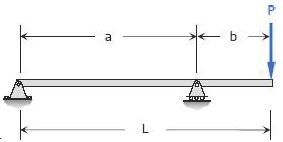
Elastic Curve

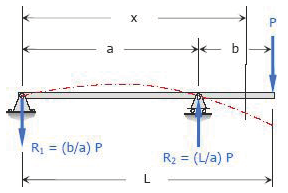
For the beam loaded as shown above, determine (a) the deflection and slope under the load P and (b) the maximum deflection between the supports.

∑ M_R2 = 0

a R₁ = b P

R₁ = b / a P

∑ M_R1 = 0

a R₂ = P L

R₂ = ( L / a ) P

E I y'' = - ( b / a ) P x + ( L / a ) P < x - a >

E I y' = - ( b / 2 a ) P x² + ( L / 2 a ) P < x - a >² + C₁

E I y = - ( b / 6a ) P x³ + ( L / 6 a ) P < x - a >³ + C₁ x + C₂

At x = 0, y = 0, therefore C2 = 0

At x = a, y = 0

0 = -[ b / (6 a) ] P a³ + a C₁

C₁ = ( a b / 6 ) P

Therefore,

E I y' = - ( b / 2a ) P x² + ( L / 2 a ) P < x - a >² + ( a b ) / 6 P

E I y = - ( b / 6a ) P x³ + ( L / 6 a ) P < x - a >³ + ( a b ) / 6 P x

Part (a): Slope and deflection under the load P

Slope under the load P: (note x = a + b = L)

E I y' = - ( b / 2a ) P ( a + b )² + ( ( a + b ) / ( 2 a) ) P b² + ( a b ) / 6 P

E I y' = - ( b / 2a ) P ( a^2 + 2 a b + b^2 ) + ( a b^2 + b^3 ) / ( 2 a ) P + ( a b ) / 6 P

E I y' = - ( a b / 2 ) P - b² P - b³ / ( 2 a ) P + b² / 2 P b³/ ( 2 a ) P + ( a b ) /6 P

E I y' = - 0.5 b² P - 0.333 a b P

E I y' = - ( 1/ 6) b ( 3 b + 2 a ) P

E I y' = - ( 1/ 6) b [ 2 ( a + b ) + b ] P

E I y' = - ( 1/ 6) b ( 2 L + b ) P

Deflection under the load P: (note x = a + b = L)

E I y = - b / ( 6 a ) P ( a + b )³ + ( a + b ) / ( 6 a ) P ( b³) + ( a b ) / 6 P ( a + b )

E I y = - b / ( 6 a ) P ( a³ + 3 a² b + 3 a b + b³) + ( a b³ + b⁴⁾ / ( 6 a ) P + ( a b ) / 6 ( P ( a + b ) )

E I y = - ( a² b ) / 6 P - ( a b²) / 2 P - b³ / 2 P - b⁴ / ( 6 a) P + b³ / 6 P + b⁴ / ( 6 a) P + ( a² b ) / 6 P + ( a b² ) / 6 P

E I y = - ( 1 /3 ) a b² P - ( 1 / 3 ) b³ P

E I y = - ( 1 /3 ) ( a + b ) b² P

E I y = - ( 1 /3 ) L b² P

Part (b): Maximum deflection between the supports The maximum deflection between the supports will occur at the point

where y' = 0.

E I y' = - b / ( 2 a ) P x² + L / ( 2 a ) P < x - a >² + ( a b ) / 6 P

At y' = 0, < z - a > do not exist thus,

0 = - b / ( 2 a ) P x² + ( a b ) /6 P

x² = 0.333 a²

x = 1 / ( 3)^1/2 a

at: 1 / ( 3)^1/2 a,

E I y_max = - b / ( 6 a ) P ( 1 / ( 3)^1/2 a )³ + ( ( a b ) / 6 ) P ( 1 / ( 3)^1/2 a )

E I y_max = - ( a² b ) / ( 6 ( 3 )^1/2 ) P + ( a² b ) / ( 6 ( 3 )^1/2 ) P

E I y_max = ( a² b ) / ( 6 ( 3 )^1/2 ) P ( - 1 / 3 + 1 )

E I y_max = ( a² b ) / ( 6 ( 3 )^1/2 ) P ( 2 / 3 )

E I y_max = ( a² b ) / ( 9 ( 3 )^1/2 ) P

Related:

• Double Integration Method for Beam Deflections
• Double Integration Method Example 1 Simply Supported Beam of Length L with Concentrated Load at Mid Span
• Double Integration Method Example 3 Proof Cantilevered Beam
• Double Integration Method Example 4 Proof Simply Supported Beam of Length L with Partial Distributed Load

Reference:

• Dr. ZM Nizam Lecture Notes
• Shingley Machine Design, 4-3 "Deflection Due to Bending"
• Beam Deflection by Integration Lecture Presentation Paul Palazolo, University of Memphis,
• Beam Deflections Using Double integration, Steven Vukazich, San Jose University