Related Resources: calculators
Solid with Spherical Inclusions Effective Thermal Conductivity Equation and Calculator
Heat Transfer Engineering
Thermodynamics
Solid with Spherical Inclusions Effective Thermal Conductivity Equation and Calculator
Effective thermal conductivity of a two-phase system
Preview: Solid with Spherical Inclusions Effective Thermal Conductivity Calculator
Construction of two systems that both contain within a spherical region of radius R' (a) the "true" system, a medium with thermal conductivity ko, in which there are embedded n tiny spheres of thermal conductivity k, and radius R; and (b) an "equivalent" system, which is a continuum, with an effective thermal conductivity keff. Both of these systems are placed in a temperature gradient A, and both are surrounded by a medium with thermal conductivity ko.
Eq. 1
Tr = T + [ 1 - ( ( keff - ko ) / ( keff + 2 · ko ) · ( R / r ) 3 ] · A · r · cos (Θ)
Where:
T = Initial Temperature (K)
keff = Effective Thermal Conductivity (J/ft s K)
ko = Thermal Conductivity of Solid (J/ft s K)
R = Effective Radius (ft)
r = Distance from Center (ft)
A = Temperature Gradient (K/ft)
Θ = Position of Substance from Center (rad)
Tr = Temperature at R (K)
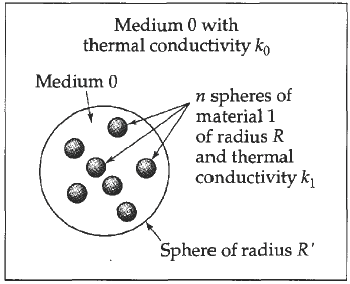
Figure 1a
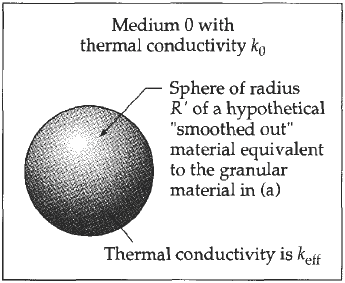
Figure 2a
Figures 1a and 1b thought experiment used by Maxwell to get the thermal conductivity of a composite solid: (a) the "true" discrete system, and (b) the "equivalent" continuum system.
Source:
- Bird, R.B., Stewart, W.E. and Lightfoot, E.N. (2002). Transport Phenomena (Second Ed.). John Wiley & Sons, Chapter: 11, Page: 371.
Related:
- Grashof Number (GR) The Grashof number is used to determine the heat transfer coefficient under free convection conditions. It is basically a ratio between the buoyancy forces and viscous forces.
- Hemispherical Emissivities of Various Surfaces Table - emissivities of various surfaces at several wavelengths and temperatures.
- Heat Loss through a Double-Pane Window Equation and Calculator
- Conductive Heat Transfer Large Plane Equation and Calculator
- Conductive Heat Transfer of a Spherical Layer Equation and Calculator
- Heat Loss from a Pipe
- Heat Loss through a Insulated Pipe Equation and Calculator
- Convective Heat Transfer Coefficients Table Chart
- Emissivity of Surface Table
- Steady State Fenestration Heat Energy Flow and Loss
- Fourier's Law for Insulation Formula